
In a similar way, the Fourier image can be re-transformed to the F(0,0) represents the DC-component of the image which corresponds to the average brightness and F(N-1,N-1) represents the highest frequency. The basis functions are sine and cosine waves with increasing frequencies, i.e. Spatial image with the corresponding base function and summing the The equation can be interpreted as: the value ofĮach point F(k,l) is obtained by multiplying the Term is the basis function corresponding to each point F(k,l) in Where f(a,b) is the image in the spatial domain and the exponential the image in the spatial and Fourier domain are of theįor a square image of size N×N, the two-dimensional DFT is given by: Number of frequencies corresponds to the number of pixels in the spatialĭomain image, i.e. Which is large enough to fully describe the spatial domain image. The DFT is the sampled Fourier Transform and therefore does notĬontain all frequencies forming an image, but only a set of samples Image analysis, image filtering, image reconstruction and imageĪs we are only concerned with digital images, we will restrict thisĭiscussion to the Discrete Fourier Transform (DFT). The Fourier Transform is used in a wide range of applications, such as Particular frequency contained in the spatial domain image. In the Fourier domain image, each point represents a Represents the image in the Fourier or frequencyĭomain, while the input image is the spatial domainĮquivalent.
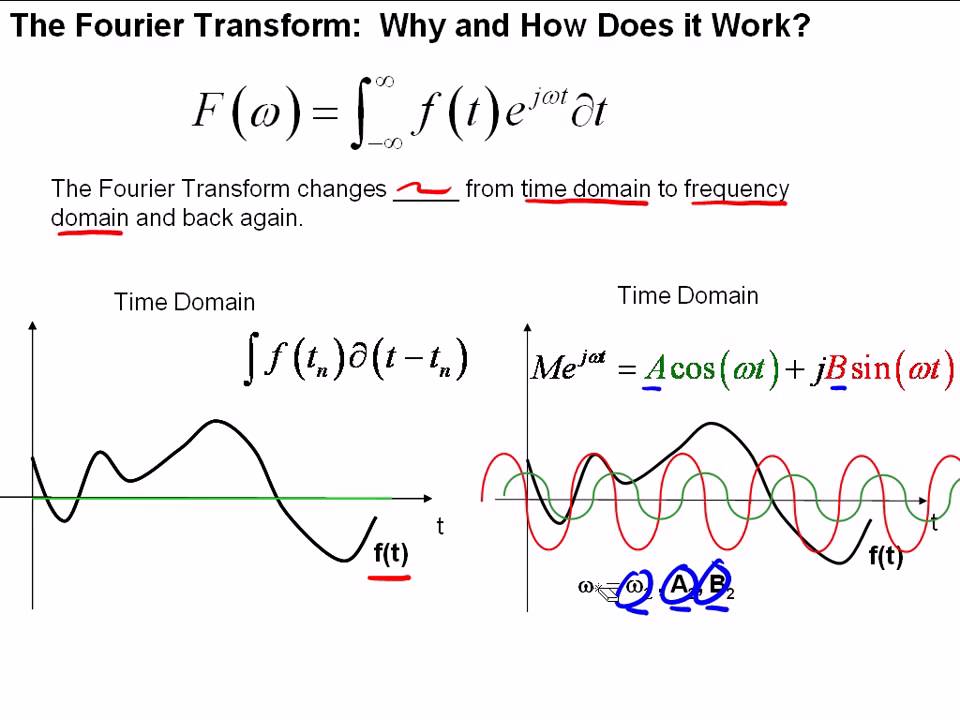
Important image processing tool which is used to decompose an image Common Names: Fourier Transform, Spectral Analysis, Frequency Analysis


 0 kommentar(er)
0 kommentar(er)
